Optimal choice of discretization points
(Prof. Dr. Anke Pohl)
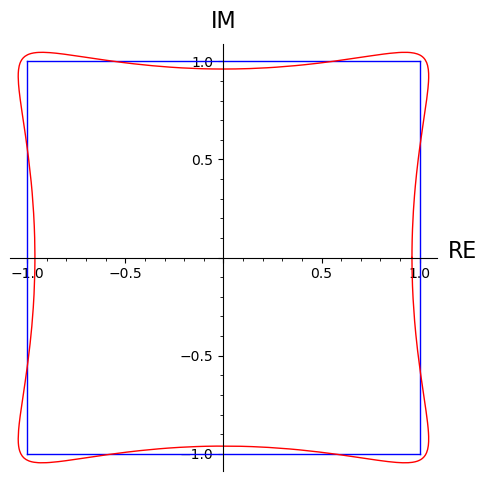
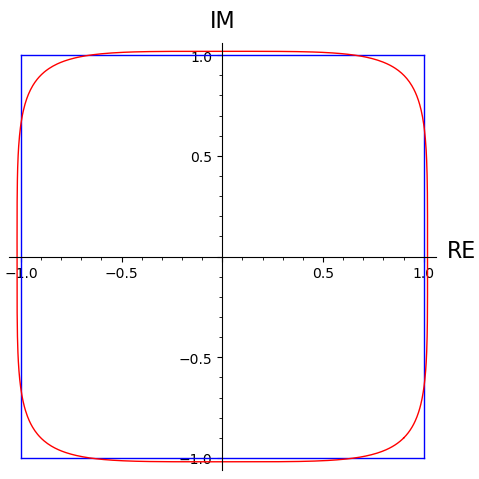
With complex Fourier series one can create many two-dimensional "one-line drawings". The selection of the collocation points for the approximating, finite Fourier series (the Fourier polynomial) has a great influence on the beauty of a drawing. Below you can see two approximations of the unit square by Fourier polynomials of order 5, which only differ in the choice of the collocation points. The difference is obvious! The aim of this project is to investigate which choices of support points are good for a simple figure (e.g. a square, a triangle, etc.).
Basic knowledge in the fields of analysis (calculus 1 & 2) and linear algebra is recommended,
in particular a certain familiarity with Fourier series, and some basic experience with programming. The duration of the project work within the working group should be at least four weeks. Part of this project is a final paper and a presentation in a seminar.